Home | Audio | DIY | Guitar | iPods | Music | Brain/Problem Solving | Links| Site Map
This work is licensed under a Creative Commons License.
Binary Numeral System
Representation
A binary number can be represented by any sequence of bits (binary digits), which in turn may be represented by any mechanism capable of being in two mutually exclusive states. The following sequences of symbols could all be interpreted as the binary numeric value of 667:
1 0 1 0 0 1 1 0 1 1 | - | - - | | - | | x o x o o x x o x x y n y n n y y n y y A binary clock might use LEDs to express binary values. In this clock, each column of LEDs shows a binary-coded decimal numeral of the traditional sexagesimal time.
A binary clock might use LEDs to express binary values. In this clock, each column of LEDs shows a binary-coded decimal numeral of the traditional sexagesimal time.The numeric value represented in each case is dependent upon the value assigned to each symbol. In a computer, the numeric values may be represented by two different voltages; on a magnetic disk, magnetic polarities may be used. A "positive", "yes", or "on" state is not necessarily equivalent to the numerical value of one; it depends on the architecture in use.
In keeping with customary representation of numerals using Arabic numerals, binary numbers are commonly written using the symbols 0 and 1. When written, binary numerals are often subscripted, prefixed or suffixed in order to indicate their base, or radix. The following notations are equivalent:
- 100101 binary (explicit statement of format)
- 100101b (a suffix indicating binary format)
- 100101B (a suffix indicating binary format)
- bin 100101 (a prefix indicating binary format)
- 1001012 (a subscript indicating base-2 (binary) notation)
- %100101 (a prefix indicating binary format)
- 0b100101 (a prefix indicating binary format, common in programming languages)
The final notation is used if converting using Google. For example searching 5 in base 2 in google results in 5 = 0b101.
When spoken, binary numerals are usually read digit-by-digit, in order to distinguish them from decimal numbers. For example, the binary numeral 100 is pronounced one zero zero, rather than one hundred, to make its binary nature explicit, and for purposes of correctness. Since the binary numeral 100 is equal to the decimal value four, it would be confusing to refer to the numeral as one hundred. Counting in the Binary system is a complex thing to do, but if you follow these steps it may become easier:
Binary Arithmetic
For some important aspects of Internet engineering, most notably IP Addressing, an understanding of binary arithmetic is critical. Many strange-looking decimal numbers can only be understood by converting them (at least mentally) to binary.
All digital computers represent data as a collection of bits. A bit is the smallest possible unit of information. It can be in one of two states - off or on, 0 or 1. The meaning of the bit, which can represent almost anything, is unimportant at this point. The thing to remember is that all computer data - a text file on disk, a program in memory, a packet on a network - is ultimately a collection of bits.
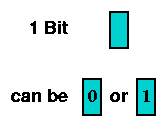
If one bit has two different states, how many states do two bits have? The answer is four. Likewise, three bits have eight states. For example, if a computer display had eight colors available, and you wished to select one of these to draw a diagram in, three bits would be sufficient to represent this information. Each of the eight colors would be assigned to one of the three-bit combinations. Then, you could pick one of the colors by picking the right three-bit combination.
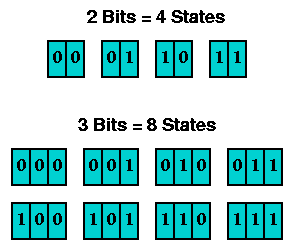
A common and convenient grouping of bits is the byte or octet, composed of eight bits. If two bits have four combinations, and three bits have eight combinations, how many combinations do eight bits have? If you don't want to write out all the possible byte patterns, just multiply eight twos together - one two for each bit. Two times two is four, so the number of combinations of two bits is four. Two times two times two is eight, so the number of combinations of three bits is eight. Do this eight times - or just compute two to the eighth power - and you discover that a byte has 256 possible states.

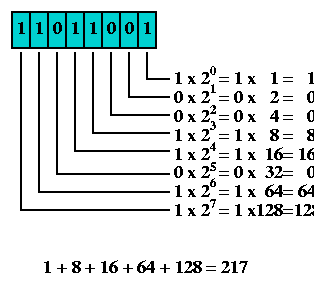
Obviously, if a byte has 256 possible states, its exact state can be represented by a number from 1 to 256. However, since zero is a very important number, a byte is more typically represented by a number from 0 to 255. This is very common, and with bit pattern 00000000 representing zero, and bit pattern 11111111 representing 255. The numbers matching these two patterns, and everything in between, can be computed by assigning a weight to each bit, multiplying each bit's value (0 or 1) by its weight, and then adding the totals. For example, here's how 217 is represented as 11011001 in binary:
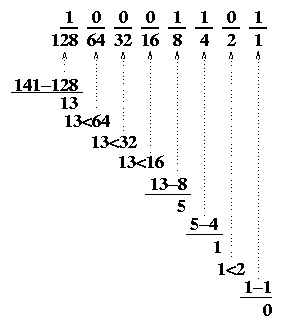
To convert a number from decimal to binary, begin at leftmost bit position (128). If the number is larger than or equal to the bit's weight, write a 1 in the bit position, subtract the bit's weight from the number, and continue with the difference. If the number is less than the bit's weight, write a 0 in the bit position and continue without any subtraction. Here's an illustration of converting 141 to binary:
There is a simpler way to convert bytes back and forth between binary and decimal; akin to memorizing multiplication tables. The byte can split into two four-bit halves, each half called a nibble. Memorize the decimal values for the high nibble (they're just the multiples of 16). The low nibble is trivial. Every number between 0 and 255 is the sum of one of the high nibble values and one of the low nibble values. Write the high nibble next to the low nibble, and you have the byte value in binary. Conversely, an eight-bit binary byte can be split in half, each nibble converted to decimal and two decimal numbers added together.
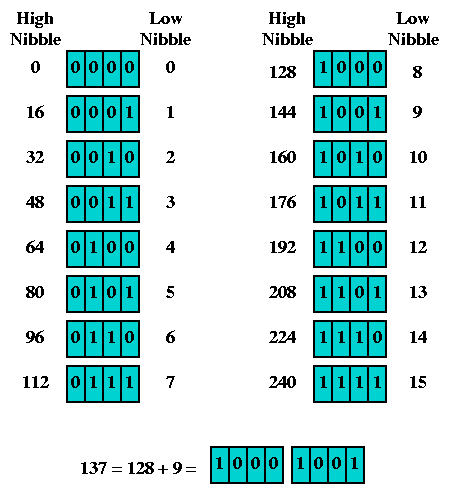
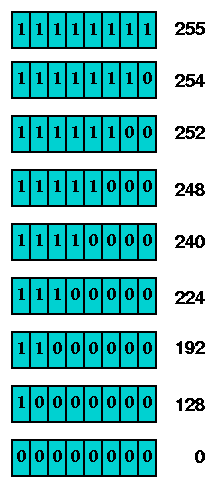
The most common bit patterns in Internet engineering are those with a string of one bits, followed by a string of zero bits. Here are all such bytes, along with their decimal representation, computed just like the example using 217.
Counting in binary
| Decimal | Binary |
|---|---|
| 0 | 0 |
| 1 | 1 |
| 2 | 10 |
| 3 | 11 |
| 4 | 100 |
| 5 | 101 |
| 6 | 110 |
| 7 | 111 |
| 8 | 1000 |
| 9 | 1001 |
| 10 | 1010 |
Counting in binary is similar to counting in any other number system. Beginning with a single digit, counting proceeds through each symbol, in increasing order. Decimal counting uses the symbols 0 through 9, while binary only uses the symbols 0 and 1.
When the symbols for the first digit are exhausted, the next-higher digit (to the left) is incremented, and counting starts over at 0. In decimal, counting proceeds like so:
- 000, 001, 002, ... 007, 008, 009, (rightmost digit starts over, and next digit is incremented)
- 010, 011, 012, ...
- ...
- 090, 091, 092, ... 097, 098, 099, (rightmost two digits start over, and next digit is incremented)
- 100, 101, 102, ...
After a digit reaches 9, an increment resets it to 0 but also causes an increment of the next digit to the left. In binary, counting is the same except that only the two symbols 0 and 1 are used. Thus after a digit reaches 1 in binary, an increment resets it to 0 but also causes an increment of the next digit to the left:
- 0000,
- 0001, (rightmost digit starts over, and next digit is incremented)
- 0010, 0011, (rightmost two digits start over, and next digit is incremented)
- 0100, 0101, 0110, 0111, (rightmost three digits start over, and the next digit is incremented)
- 1000, 1001, ...
Since binary is a base-2 system, each digit represents an increasing power of 2, with the rightmost digit representing 20, the next representing 21, then 22, and so on. To determine the decimal representation of a binary number simply take the sum of each of the product binary digits with the power of 2 which they represent. For example, the binary number:
100101
is converted to decimal form by:
[(1) × 25] + [(0) × 24] + [(0) × 23] + [(1) × 22] + [(0) × 21] + [(1) × 20] =
[1 × 32] + [0 × 16] + [0 × 8] + [1 × 4] + [0 × 2] + [1 × 1] = 37
To create higher numbers, additional digits are simply added to the left side of the binary representation.
Binary arithmetic
Arithmetic in binary is much like arithmetic in other numeral systems. Addition, subtraction, multiplication, and division can be performed on binary numerals.
Addition
The circuit diagram for a binary half adder, which adds two bits together, producing sum and carry bits.
The simplest arithmetic operation in binary is addition. Adding two single-digit binary numbers is relatively simple, using a form of carrying:
- 0 + 0 ? 0
- 0 + 1 ? 1
- 1 + 0 ? 1
- 1 + 1 ? 0, carry 1 (since 1 + 1 = 0 + 1 × 10 in binary)
Adding two "1" digits produces a digit "0", while 1 will have to be added to the next column. This is similar to what happens in decimal when certain single-digit numbers are added together; if the result equals or exceeds the value of the radix (10), the digit to the left is incremented:
- 5 + 5 ? 0, carry 1 (since 5 + 5 = 0 + 1 × 10)
- 7 + 9 ? 6, carry 1 (since 7 + 9 = 6 + 1 × 10)
This is known as carrying. When the result of an addition exceeds the value of a digit, the procedure is to "carry" the excess amount divided by the radix (that is, 10/10) to the left, adding it to the next positional value. This is correct since the next position has a weight that is higher by a factor equal to the radix. Carrying works the same way in binary:
1 1 1 1 1 (carried digits) 0 1 1 0 1 + 1 0 1 1 1 ------------- = 1 0 0 1 0 0In this example, two numerals are being added together: 011012 (1310) and 101112 (2310). The top row shows the carry bits used. Starting in the rightmost column, 1 + 1 = 102. The 1 is carried to the left, and the 0 is written at the bottom of the rightmost column. The second column from the right is added: 1 + 0 + 1 = 102 again; the 1 is carried, and 0 is written at the bottom. The third column: 1 + 1 + 1 = 112. This time, a 1 is carried, and a 1 is written in the bottom row. Proceeding like this gives the final answer 1001002 (36 decimal).
When computers must add two numbers, the rule that: x xor y = (x + y) mod 2 for any two bits x and y allows for very fast calculation, as well.
A simplification for many binary addition problems is the Long Carry Method or Brookhouse Method of Binary Addition. This method is generally useful in any binary addition where one of the numbers has a long string of “1” digits. For example the following large binary numbers can be added in two simple steps without multiple carries from one place to the next.
1 1 1 1 1 1 1 1 (carried digits) (Long Carry Method) 1 1 1 0 1 1 1 1 1 01 1 101 1 1 1 10 + 1 0 1 0 1 1 0 0 1 1 Versus: + 1 010 1 1 0 011 ----------------------- + 1 0 0 0 1 0 0 0 0 0 0 sum of crossed out digits = 1 0 0 0 1 1 1 0 0 0 1 ----------------------- now add remaining digits 1 1 0 0 1 1 1 0 0 0 1In this example, two numerals are being added together: 1 1 1 0 1 1 1 1 1 02 (95810) and 1 0 1 0 1 1 0 0 1 12 (69110). The top row shows the carry bits used. Instead of the standard carry from one column to the next, the lowest place-valued "1" with a "1" in the corresponding place value beneath it may be added and a "1" may be carried to one digit past the end of the series. These numbers must be crossed off since they are already added. Then simply add that result to the uncanceled digits in the second row. Proceeding like this gives the final answer 1 1 0 0 1 1 1 0 0 0 12 (164910).
Subtraction
Subtraction works in much the same way:
- 0 - 0 ? 0
- 0 - 1 ? 1, borrow 1
- 1 - 0 ? 1
- 1 - 1 ? 0
Subtracting a "1" digit from a "0" digit produces the digit "1", while 1 will have to be subtracted from the next column. This is known as borrowing. The principle is the same as for carrying. When the result of a subtraction is less than 0, the least possible value of a digit, the procedure is to "borrow" the deficit divided by the radix (that is, 10/10) from the left, subtracting it from the next positional value.
* * * * (starred columns are borrowed from) 1 1 0 1 1 1 0 - 1 0 1 1 1 ---------------- = 1 0 1 0 1 1 1Subtracting a positive number is equivalent to adding a negative number of equal absolute value; computers typically use two's complement notation to represent negative values. This notation eliminates the need for a separate "subtract" operation. Using two's complement notation subtraction can be summarized by the following formula:
A - B = A + not B + 1
For further details, see two's complement.
Multiplication
Multiplication in binary is similar to its decimal counterpart. Two numbers A and B can be multiplied by partial products: for each digit in B, the product of that digit in A is calculated and written on a new line, shifted leftward so that its rightmost digit lines up with the digit in B that was used. The sum of all these partial products gives the final result.
Since there are only two digits in binary, there are only two possible outcomes of each partial multiplication:
- If the digit in B is 0, the partial product is also 0
- If the digit in B is 1, the partial product is equal to A
For example, the binary numbers 1011 and 1010 are multiplied as follows:
1 0 1 1 (A) × 1 0 1 0 (B) --------- 0 0 0 0 ? Corresponds to a zero in B + 1 0 1 1 ? Corresponds to a one in B + 0 0 0 0 + 1 0 1 1 --------------- = 1 1 0 1 1 1 0Binary numbers can also be multiplied with bits after a binary point:
1 0 1.1 0 1 (A) (5.625 in decimal) × 1 1 0.0 1 (B) (6.25 in decimal) ------------- 1 0 1 1 0 1 ? Corresponds to a one in B + 0 0 0 0 0 0 ? Corresponds to a zero in B + 0 0 0 0 0 0 + 1 0 1 1 0 1 + 1 0 1 1 0 1 ----------------------- = 1 0 0 0 1 1.0 0 1 0 1 (35.15625 in decimal)See also Booth's multiplication algorithm.
Division
Binary division is again similar to its decimal counterpart:
Here, the divisor is 1012, or 5 decimal, while the dividend is 110112, or 27 decimal. The procedure is the same as that of decimal long division; here, the divisor 1012 goes into the first three digits 1102 of the dividend one time, so a "1" is written on the top line. This result is multiplied by the divisor, and subtracted from the first three digits of the dividend; the next digit (a "1") is included to obtain a new three-digit sequence:
1 ___________ 1 0 1 ) 1 1 0 1 1 - 1 0 1 ----- 0 1 1The procedure is then repeated with the new sequence, continuing until the digits in the dividend have been exhausted:
1 0 1 ___________ 1 0 1 ) 1 1 0 1 1 - 1 0 1 ----- 0 1 1 - 0 0 0 ----- 1 1 1 - 1 0 1 ----- 1 0Thus, the quotient of 110112 divided by 1012 is 1012, as shown on the top line, while the remainder, shown on the bottom line, is 102. In decimal, 27 divided by 5 is 5, with a remainder of 2.
Bitwise operations
Though not directly related to the numerical interpretation of binary symbols, sequences of bits may be manipulated using Boolean logical operators. When a string of binary symbols is manipulated in this way, it is called a bitwise operation; the logical operators AND, OR, and XOR may be performed on corresponding bits in two binary numerals provided as input. The logical NOT operation may be performed on individual bits in a single binary numeral provided as input. Sometimes, such operations may be used as arithmetic short-cuts, and may have other computational benefits as well. For example, an arithmetic shift left of a binary number is the equivalent of multiplication by a (positive, integral) power of 2.
Conversion to and from other numeral systems
Decimal
To convert from a base-10 integer numeral to its base-2 (binary) equivalent, the number is divided by two, and the remainder is the least-significant bit. The (integer) result is again divided by two, its remainder is the next most significant bit. This process repeats until the result of further division becomes zero.
Conversion from base-2 to base-10 proceeds by applying the preceding algorithm, so to speak, in reverse. The bits of the binary number are used one by one, starting with the most significant bit. Beginning with the value 0, repeatedly double the prior value and add the next bit to produce the next value. This can be organized in a multi-column table. For example to convert 100101011012 to decimal:
| Prior value | × 2 + | Next Bit | Next value |
|---|---|---|---|
| 0 | × 2 + | 1 | = 1 |
| 1 | × 2 + | 0 | = 2 |
| 2 | × 2 + | 0 | = 4 |
| 4 | × 2 + | 1 | = 9 |
| 9 | × 2 + | 0 | = 18 |
| 18 | × 2 + | 1 | = 37 |
| 37 | × 2 + | 0 | = 74 |
| 74 | × 2 + | 1 | = 149 |
| 149 | × 2 + | 1 | = 299 |
| 299 | × 2 + | 0 | = 598 |
| 598 | × 2 + | 1 | = 1197 |
The result is 119710. This method is an application of the Horner scheme.
| Binary | 1 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 1 | 0 | 1 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Decimal | 1×210 + | 0×29 + | 0×28 + | 1×27 + | 0×26 + | 1×25 + | 0×24 + | 1×23 + | 1×22 + | 0×21 + | 1×20 = | 1197 |
Hexadecimal
Binary may be converted to and from hexadecimal somewhat more easily. This is because the radix of the hexadecimal system (16) is a power of the radix of the binary system (2). More specifically, 16 = 24, so it takes four digits of binary to represent one digit of hexadecimal.
The following table shows each hexadecimal digit along with the equivalent decimal value and four-digit binary sequence:
| Hex | Dec | Binary |
|---|---|---|
| 0 | 0 | 0000 |
| 1 | 1 | 0001 |
| 2 | 2 | 0010 |
| 3 | 3 | 0011 |
| 4 | 4 | 0100 |
| 5 | 5 | 0101 |
| 6 | 6 | 0110 |
| 7 | 7 | 0111 |
| 8 | 8 | 1000 |
| 9 | 9 | 1001 |
| A | 10 | 1010 |
| B | 11 | 1011 |
| C | 12 | 1100 |
| D | 13 | 1101 |
| E | 14 | 1110 |
| F | 15 | 1111 |
To convert a hexadecimal number into its binary equivalent, simply substitute the corresponding binary digits:
- 3A16 = 0011 10102
- E716 = 1110 01112
To convert a binary number into its hexadecimal equivalent, divide it into groups of four bits. If the number of bits isn't a multiple of four, simply insert extra 0 bits at the left (called padding). For example:
- 10100102 = 0101 0010 grouped with padding = 5216
- 110111012 = 1101 1101 grouped = DD16
To convert a hexadecimal number into its decimal equivalent, multiply the decimal equivalent of each hexadecimal digit by the corresponding power of 16 and add the resulting values:
- C0E716 = (12 × 163) + (0 × 162) + (14 × 161) + (7 × 160) = (12 × 4096) + (0 × 256) + (14 × 16) + (7 × 1) = 49,38310
Octal
Binary is also easily converted to the octal numeral system, since octal uses a radix of 8, which is a power of two (namely, 23, so it takes exactly three binary digits to represent an octal digit). The correspondence between octal and binary numerals is the same as for the first eight digits of hexadecimal in the table above. Binary 000 is equivalent to the octal digit 0, binary 111 is equivalent to octal 7, and so forth.
| Octal | Binary |
|---|---|
| 0 | 000 |
| 1 | 001 |
| 2 | 010 |
| 3 | 011 |
| 4 | 100 |
| 5 | 101 |
| 6 | 110 |
| 7 | 111 |
Converting from octal to binary proceeds in the same fashion as it does for hexadecimal:
And from binary to octal:
And from octal to decimal:
a) For (X) above add.
b) For (X) above subtract.
c) For (X) above multiply.
d) For (X) above divide.
e) Convert all of the base 2 answers in set a) above to base 10
f)
Convert all of the base 2 answers in set b) above to hexadecimal
Convert the following base 10 numbers to base 2:
Home | Audio | DIY | Guitar | iPods | Music | Links | Brain and Problem Solving | Site Map | Contact